An official website of the United States government
 United States Department of Labor
United States Department of Labor
The National Compensation Survey (NCS) is an establishment-based survey that collects data on employer costs for employee compensation and incidence and details of employer-sponsored benefits. The calculation details for the Employment Cost Index (ECI), Employer Costs for Employee Compensation (ECEC) and Employee Benefits are covered in this section.
The ECI is a measure of the change in the employer costs of labor, independent of the influence of employment shifts among occupations and industry categories. The total compensation series include changes in wages and salaries and in employer costs for employee benefits. The ECI calculates indexes of total compensation, wages and salaries, and benefits separately for all civilian workers in the United States (as defined by the NCS), for private industry workers, and for workers in state and local government. For all of these categories, the ECI calculates the same measures by occupational group, industry group, and worker and establishment characteristics. Seasonally adjusted series are calculated as well.
The ECI is a modified Laspeyres index (that is, an index reflecting the change in labor costs over time), for which the basic computational framework is the standard formula for an index number with fixed index weights, modified by special statistical conditions and accounting for sampling methodology.
An index number for the ECI is a weighted average of the cumulative average wage changes within each of the ECI basic cells, with “wage bills” serving as the fixed weights. For benefit costs, the index number is a weighted average of the cumulative average benefit costs within each of the ECI basic cells, with “benefit bills” serving as the fixed weights. A basic cell for the ECI is composed of wage (or benefit) data from a narrowly defined set of workers, sorted by ownership sector, industry, and occupational groups in which they are employed. The ECI cell structure sorts the industry codes into 1 of 3 ownership sectors: private, state government, or local government. Workers within private establishments are sorted into 1 of 59 industry categories that are defined primarily by three-digit industry codes using the 2012 North American Industry Classification System (NAICS). Workers in either state or local government are classified into 13 industry categories; the government industry categories are as broad as “all goods-producing industries” and as narrow as “hospitals.” Each of these private and government industry groups is arrayed across nine aggregate occupational groups, which are ordered numerically by their 2010 Standard Occupational Classification (SOC) codes. Altogether, there are 531 (59 × 9) private industry occupational cells and 234 (13 × 9 × 2) state and local government industry occupational cells, totaling 765 ECI basic cells.
The unweighted average wage (or benefit cost) is calculated from all workers within a sampled quote (selected job). The wage (or benefit) bill ![]() is the product of the weighted average wage (or benefit cost) of sampled quotes (selected jobs),
is the product of the weighted average wage (or benefit cost) of sampled quotes (selected jobs), ![]() , within the cell at the period
, within the cell at the period ![]() in which the wage (or benefit) bill is calculated and the number of workers represented by the cell,
in which the wage (or benefit) bill is calculated and the number of workers represented by the cell, ![]()
![]()
For the ECI, the number of workers represented by the cell is held fixed. For each basic cell, wage and benefit bills (![]() ) are computed, and the bills are updated each quarter by observed rates of change from the ECI survey sample.
) are computed, and the bills are updated each quarter by observed rates of change from the ECI survey sample.
The simplified formula for a basic cell is:
![]()
where
It is the index at period t,
![]() is the estimated wage (or benefit) bill for the ith cell, and
is the estimated wage (or benefit) bill for the ith cell, and
![]() is the multiplicatively accumulated weighted average wage (or benefit cost) change in the ith cell from time 0 (the period the wage or benefit bill is calculated) to time t (the current quarter).
is the multiplicatively accumulated weighted average wage (or benefit cost) change in the ith cell from time 0 (the period the wage or benefit bill is calculated) to time t (the current quarter). ![]() projects the weighted average wage (or benefit cost) level for the cell forward to the current quarter.
projects the weighted average wage (or benefit cost) level for the cell forward to the current quarter.
Note that ![]() can be written as
can be written as ![]()
where
![]() is the ratio of the current-quarter weighted average wage (or average benefit costs) in the cell to the previous-quarter weighted average wage (or average benefit costs) in the cell, both calculated in the current quarter from matched-sampled quotes. Using only matched quotes in the ratio eliminates the inclusion of wage (or benefit cost) changes that might be caused by workers reassigned between jobs within establishments or changes of jobs sampled in the survey. That is, the ECI sample tracks changes in wages (or benefit cost) within establishment jobs, not by individual workers of the establishment. The sample quote weights are applied to compute the cell-weighted averages.
is the ratio of the current-quarter weighted average wage (or average benefit costs) in the cell to the previous-quarter weighted average wage (or average benefit costs) in the cell, both calculated in the current quarter from matched-sampled quotes. Using only matched quotes in the ratio eliminates the inclusion of wage (or benefit cost) changes that might be caused by workers reassigned between jobs within establishments or changes of jobs sampled in the survey. That is, the ECI sample tracks changes in wages (or benefit cost) within establishment jobs, not by individual workers of the establishment. The sample quote weights are applied to compute the cell-weighted averages.
All wage and benefit indexes are computed from the following data:
Computation of the index for a calendar quarter involves five principal steps:
Computations for the occupational and industry group indexes follow the same procedures as those for the overall indexes, except for summation. For example, for an index for a broad occupational group, the wage (or benefit) bills are summed across all cells, which are a subset of that occupational group, with indexes for industry groups calculated analogously.
Fixed employment weights are used each quarter to calculate aggregate indexes for civilian, private, and state and local government. These fixed weights are also used to derive all of the industry and occupation series indexes, see Introducing 2012 fixed employment weights for the ECI.
Computation procedures for measures of change in the regional, union and nonunion, and excluding-incentive workers indexes differ from those of the national wage and benefit indexes because the sample is not large enough to hold the wage and benefit bills constant at the level of detail of the indexes for larger samples. For these indexes, the prevailing distribution in the sample in the previous quarter (for example, between union and nonunion attributes within each ownership–industry–occupation cell of the previous quarter) is used to apportion the previous-quarter wage (or benefit) bill in that cell (for example, between the union and nonunion indexes) each quarter. The portion of the wage (or benefit) bill assigned to the union index is then adjusted by the percent change in the union wages (or benefit costs) in the cell, and similarly for the nonunion index. Therefore, the relative employment of the union index in each cell is not held constant over time and will likely change each quarter as the sample changes as well as actual changes in the employment distributions across these attributes. Because the weights of the region, union, and time-paid workers indexes are allowed to vary over time, these indexes are not strictly comparable to the aggregate, industry, occupation, and metropolitan area indexes.
ECI seasonal adjustment
Over the course of a year, rates of change in the cost of wages and benefits, as measured in the ECI, reflect events that follow a more or less regular pattern. These events include expansions and contractions of economic activity that occur in specific periods of the year, such as increased work in the construction industry during warm weather or changes in education stemming from new contracts associated with the beginning of the new school year. Such regular patterns in an economic time series typically are referred to as seasonal effects. The process of estimating and removing these effects from an economic series is called seasonal adjustment. Seasonal adjustment makes it easier for analysts to observe changes in data exclusive of seasonal effects. Economists and other researchers are particularly interested in observing cyclical and long-run movements of economic series to gain a better understanding of the economic behavior of various sectors of the economy.
In evaluating changes in a seasonally adjusted series, it is important to note that seasonal adjustment is an approximation based on past experience. Seasonally adjusted data have a similar margin of error as the original data on which they are based; therefore, the standard errors of the original (not seasonally adjusted) series could be used to assess the approximate precision of the corresponding seasonally adjusted estimates.
Seasonal adjustment is performed with the X-13ARIMA-SEATS program developed by staff of the Statistical Research Division of the U.S. Census Bureau. The X-13ARIMA-SEATS program includes enhancements to both the X-11 variant of the Census Method II seasonal adjustment program and the X-11 ARIMA (Autoregressive Integrated Moving Average) program developed by Statistics Canada. For a definition and explanatory information on ARIMA, see The X-13ARIMA-SEATS Seasonal Adjustment Program and The X-II-ARIMA Seasonal Adjustment Method.
ECI series are seasonally adjusted by either a direct or an indirect method. In the direct method, an original (or unadjusted) index is divided by its seasonal factor estimated from X-13ARIMA-SEATS. In the indirect method, also called composite seasonal adjustment, the seasonally adjusted index is calculated as a weighted sum of seasonally adjusted index components, where the weights are derived from the index weights.
Indexes at comparatively low levels of aggregation, such as the construction wage index, are adjusted by the direct method; that is, dividing the index by its seasonal factor. Higher level aggregate indexes, such as civilian wages and salaries, are generally seasonally adjusted by the indirect method, a weighted sum of seasonally adjusted component indexes, where the weights sum to 1.0. Industry and occupational series that are seasonally adjusted by the indirect method are based on industry and occupational components, respectively.
At the beginning of each calendar year, seasonal adjustment factors are estimated. The seasonal factors for the directly adjusted series for the entire year are published. Seasonally adjusted estimates are revised each year, for a 5-year period, based on the latest year of data available. NCS publishes these revised seasonally adjusted series, directly and indirectly adjusted, annually. For additional information see Employment Cost Index: Annual Seasonal Adjustment Process.
The ECEC measures the average costs to employers for wages and salaries, and benefits, per employee hour worked. The series provides data on employer costs per hour worked for total compensation, wages and salaries, total benefits, and the following benefits:
Cost data are presented both in dollar amounts and as percentages of total compensation and published quarterly.
The ECEC series provides an average cost across all workers. Eligible workers with access to employer-sponsored benefits who do not participate are also included in the calculation. That is, the average cost includes workers for whom the employer incurred a compensation cost and those for whom no cost was incurred.
The ECEC uses current employment weights (as opposed to fixed employment weights used in the ECI) to reflect the changing composition of today’s labor force to calculate cost levels. The employment weights are derived from two BLS programs: the Quarterly Census of Employment and Wages (QCEW) and the Current Employment Statistics (CES). Combined, these programs provide the appropriate industry coverage and currency of data needed to benchmark (post-stratify) employment weights for the ECEC series.
In most instances, private industry employment weights used in the ECEC are total employment estimates for two-digit industry groups, such as utilities (NAICS 22) or wholesale trade (NAICS 42). In a few cases, the employment weights associated with more detailed industrial categories are used. Among such categories are the four-digit NAICS categories elementary and secondary schools (6111), junior colleges (6122), colleges and universities (6133), and the six-digit NAICS category aircraft manufacturing (336411). For state and local governments, a more aggregated level reflecting the level of detail published by the Current Employment Statistics (CES) program is typically used.
The ECEC estimates of the percentage of total compensation are calculated from unrounded estimates of hourly employer costs and then the percentages are rounded to the first decimal place. This method provides the most precise estimates of the percentage of total compensation; estimates calculated from published cost estimates may differ slightly from those calculated from unpublished unrounded cost estimates.
The formula for the mean hourly cost c for domain ![]() is:
is:
![]()
where
![]() is the domain of interest (such as all manufacturing workers)
is the domain of interest (such as all manufacturing workers)
![]() is the final quote weight for quote
is the final quote weight for quote ![]() , calculated as described earlier, with one additional factor included to account for changes in the employment distribution,
, calculated as described earlier, with one additional factor included to account for changes in the employment distribution,
and ![]() is the mean hourly cost
is the mean hourly cost ![]() for quote
for quote ![]() .
.
The formula for the mean hourly cost c as a percentage of total compensation is:
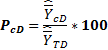
where
![]() is the mean hourly cost c for domain
is the mean hourly cost c for domain ![]() , as before, and
, as before, and
![]() is the mean hourly cost for total compensation for domain
is the mean hourly cost for total compensation for domain ![]() .
.
When respondents do not provide all the data needed, a procedure for assigning plausible values for the missing values is used. The process is explained in the section Weighting, nonresponse adjustment, imputation, and benchmarking.
The NCS collects and publishes data annually on the incidence of employer-provided benefits and on the key provisions (terms) of employee benefit plans, for civilian workers, workers in private industry, and state and local government workers. The following lists the types of published benefits.
In addition, the NCS publishes data on detailed provisions of coverage in two major benefit areas: health insurance and retirement plans. Health data include medical plan provisions, such as deductibles, coinsurance, and out-of-pocket maximums, as well as details of dental, vision, and prescription drug benefits. Provisions of defined benefit and defined contribution retirement plans, such as eligibility requirements and benefit formulas, also are published. Detailed provision estimates are produced based on the initiation year (for example, the first year of participation in the NCS for the sampled establishment) of each sample group collected data via Summary Plan Description (SPD), plan summary sheets, and Summary of Benefits and Coverage (SBC).
Formula used to calculate access to benefits. The formula for the percentage of employees with access ![]() to a benefit area, such as life insurance, for domain
to a benefit area, such as life insurance, for domain ![]() is:
is:
![]()
where
![]() is the domain of interest,
is the domain of interest,
![]() is the final weight for quote
is the final weight for quote ![]() , calculated as described in the section on the calculation of ECEC estimates, and
, calculated as described in the section on the calculation of ECEC estimates, and
![]() if the worker in quote q has access to the benefit being estimated and
if the worker in quote q has access to the benefit being estimated and ![]() otherwise.
otherwise.
Formula used to calculate benefit participation. The formula for the incidence ![]() , or percentage, of employees participating in a benefit area, such as medical care, for domain
, or percentage, of employees participating in a benefit area, such as medical care, for domain ![]() is
is
![]()
where
![]() is the domain of interest,
is the domain of interest,
![]() is the final quote weight for quote
is the final quote weight for quote ![]() , calculated as described in the section on the calculation of ECEC estimates, and
, calculated as described in the section on the calculation of ECEC estimates, and
![]() is the percentage of workers in quote
is the percentage of workers in quote ![]() who are participating in benefit-area plan
who are participating in benefit-area plan ![]() .
.
Other estimates of incidence, such as the percentage of participants in a benefit area or in a subset of a benefit area, can be computed in a similar manner, such that the base includes only those workers who participate in the benefit-area plans. For example, to calculate the percentage of medical insurance participants in fee-for-service plans in domain ![]() , a ratio is calculated such that the denominator is the same as the numerator in the previous formula and the numerator is of the same form as well, except that the summation is restricted to those participants in fee-for-service plans.
, a ratio is calculated such that the denominator is the same as the numerator in the previous formula and the numerator is of the same form as well, except that the summation is restricted to those participants in fee-for-service plans.
Formula used to calculate average (mean). The formula for the average flat monthly employee contribution for medical insurance for domain ![]() is
is
![]()
where
![]() is the domain of interest,
is the domain of interest,
![]() , is the final quote weight for quote
, is the final quote weight for quote ![]() , calculated as described in the section on the calculation of ECEC estimates,
, calculated as described in the section on the calculation of ECEC estimates,
![]() is the average monthly employee contribution to plan
is the average monthly employee contribution to plan ![]() by workers in quote
by workers in quote ![]() , and
, and
![]() is the percentage of workers in quote
is the percentage of workers in quote ![]() who are participating in plan
who are participating in plan ![]() .
.
Other means, such as the average annual deductible for medical insurance, can be calculated by a similar formula. In all cases, the averages include only those workers with the provision in question.
The weighted count of workers participating in plans available to workers in the sampled occupation and establishment is calculated by multiplying the final benchmarked quote weight by the participation rate for only those plans in the quote that meet the specific conditions defined by the quote condition and the plan conditions.
![]() where
where
![]() establishment,
establishment,
![]() occupation within establishment
occupation within establishment ![]() ,
,
![]() plan in occupation q within establishment
plan in occupation q within establishment ![]() ,
,
![]() weighted plan employment of participating workers
weighted plan employment of participating workers ![]() ,
,
![]() final benchmarked quote weight for occupation
final benchmarked quote weight for occupation ![]() in establishment
in establishment ![]() ,
,
![]() ,
, ![]() , and
, and ![]() are dummy variables such that
are dummy variables such that
![]() if quote
if quote ![]() meets the condition set in the quote (row)
meets the condition set in the quote (row)
condition
![]() otherwise,
otherwise,
![]() if plan
if plan ![]() meets the condition set in the base (denominator) plan condition
meets the condition set in the base (denominator) plan condition
![]() otherwise,
otherwise,
![]() if plan
if plan ![]() meets the condition set in the additional (numerator) plan condition
meets the condition set in the additional (numerator) plan condition
![]() otherwise, and
otherwise, and
![]() percentage of workers in occupation
percentage of workers in occupation ![]() and establishment
and establishment ![]() who are participating in plan
who are participating in plan ![]() .
.
Calculation of percentiles
Percentiles of benefit provisions are calculated with data only from those workers in plans that include the provision in question. Percentile data are used to describe the distribution of a numeric value, such as a median annual deductible of $400.00 and the value $600.00 at the 90th percentile. The following percentiles p are calculated: 10, 25, 50 (median), 75, and 90.
The pth percentile is the value Qiqj, where the plan value of a quantity is for a specific benefit or a subset of a benefit area, such that
It is possible that there are no specific plan records qi for which both of these properties hold. This occurs when there exists a plan for which the WPEiqj of records whose value is less than Qiqj equals p percent of the total weighted plan employment. In that situation, the pth percentile is the average of Qiqj and the value on the record with the next-lowest value. The Qiqj values must be sorted in ascending order.
Weighting, nonresponse adjustment, imputation, and benchmarking
Participation in the NCS is voluntary; therefore, a company official may refuse to participate in the initial survey or may be unwilling or unable to update previously provided data for one or more occupations during subsequent contact. In addition, some establishments selected from the sample frame may be out of the scope for the survey or have gone out of business. To address the problems of nonresponse and missing data, the NCS adjusts the weights of the remaining establishments and imputes missing values (for example, fills in missing values with plausible values). To ensure that published compensation estimates ultimately are representative of compensation in the civilian, private industry, and state and local government sectors.
Weight adjustments and imputation are made in accordance with the following steps:
Step 1. Unit nonresponse adjustment: An establishment is considered responding if it provided information on at least one usable occupation. A selected occupation is classified as usable if the following data are present: occupational attributes (full-time or part-time schedule, union or nonunion status, and time or incentive type of pay), work schedule, and wage data. Wages account for approximately 70 percent of compensation; therefore, if wage data are not available, other data from the establishment cannot be used in calculating estimates. Without the wage data, it is not possible to create benefit-cost estimates because many benefits, such as paid leave, for example, are linked to wages.
An establishment is considered nonresponding if it refused to participate in the survey or provided neither wages and salaries, occupational classification, worker attributes, and work schedule data for any selected occupation. Establishment nonresponse during the initial interview (referred to as initiation) is addressed by introducing nonresponse adjustments that redistribute the weights of nonrespondents to responding sample units in the same industry and size class. For example, if the nonresponding establishment was in the manufacturing industry and had an employment of 350 workers, the NCS would adjust the weights of responding manufacturing establishments with 250–499 workers by a nonresponse factor calculated by dividing the sum of the product of establishment employment and sample weight for responding and nonresponding establishments by the sum of the product of establishment employment and sample weight for responding establishments.
Step 2. Quote nonresponse adjustment: Quote nonresponse is a situation in which an establishment refuses to provide any wage data for a given sampled occupation (quote). Quote nonresponse during the initial interview is addressed by an adjustment that redistributes the weights of nonresponding quotes to responding sample quotes in the same occupational group, ownership, industry, and size class. Quote nonresponse during update interview is addressed by imputation.
Step 3. Item nonresponse is a situation in which an establishment responds to the survey but is unable or unwilling to provide some or all of the benefits data, for a given sampled occupation. Item nonresponse is addressed through item imputation in certain situations. Item imputation replaces missing values for an item with values derived from establishments with similar characteristics.
For benefit estimates, items can be imputed for nonresponse at initial and subsequent data collection. For example, during the initial contact, an establishment reports wage and salary data for a sampled occupation but refuses or is unable to report whether those in the occupation receive paid vacation benefits; the NCS imputes the incidence of vacation benefits for the selected occupation on the basis of the incidence of vacation benefits among similar occupations in similar establishments.
For wages and salaries, cost data are not imputed for item nonresponse during the establishment’s initial data collection but are imputed at subsequent data collections (update). For example, if a manufacturing establishment reported wages and salaries for its full-time nonunion assembly workers during the initial collection, but not in a subsequent collection period (update), the NCS calculates the rate of change in wages and salaries of full-time nonunion workers in similar manufacturing establishments between the two collection periods, where the rate of change in wages and salaries between two collection periods is estimated from a regression model fit to establishments who reported wage data in both periods. This rate is then multiplied by the establishment reported wages and salaries, at initiation, to impute missing wages and salaries. However, if the establishment did not provide wages and salaries for full-time nonunion assembly workers at the initial collection, the NCS would perform a quote nonresponse adjustment.
Additional adjustment factors are applied to special situations that may have occurred during data collection. For example, when a sample unit is one of two establishments owned by a given company and the respondent provides data for both locations combined instead of data for the sampled unit, the weight of the sampled unit is adjusted to reflect the employment data for the sampled unit.
Step 4 Benchmarking (poststratification). The benchmark calculation is essentially the same for all NCS data products; however, the input to the calculation differs by data product. The ECI uses fixed employment weights from the QCEW and OES programs, whereas the ECEC and benefits estimates use current weights from the CES program. Benchmarking, is the process of adjusting the weight of each establishment in the survey to match the most current distribution of employment by industry.
The private industry sample also uses establishment employment size class in the benchmarking process. The NCS establishment sample is drawn from the Quarterly Census of Employment and Wages (QCEW). The QCEW and the railroad information provide employment data, but because these sources do not have current employment data, the CES is used to adjust employment. The benchmark process updates the initial establishment weights, assigned during sampling, by current employment. Establishment weights reflect employment at the time of sampling, not collection. Benchmarking ensures that survey estimates reflect the most current industry composition–employment counts in proportions consistent with the private industry, state government, and local government sectors (hereafter, ownership).
For example, 40 private industry, 10 local government, and 5 state government units in the service sector were selected from the sampling frame made up of establishments employing 200,000 private workers, 30,000 local government workers, and 10,000 state government workers. By the time of survey processing, the private service sector employment increased by 10,000 workers, or 5 percent, with no increase in employment in the service sectors of state and local government. In the absence of benchmarking, the sample would underrepresent current employment in the private industry service sector. In this example, the NCS adjusts the sample weights of the 40 service sector firms in private industry to ensure that the number of workers in establishments in the sampling frame rises to 210,000. The ownership employment counts for the private industry service sector would then reflect the current proportions of 84 percent for private industry, 12 percent for local government, and 4 percent for state government employment.
Calculating estimate reliability
Two types of errors are possible in an estimate based on a sample survey: sampling errors and nonsampling errors. Sampling errors occur because the sample makes up only a part of the population it represents. The sample used for the survey is one of a number of possible samples that could have been selected under the sample design, each producing its own estimate. A measure of the variation among sample estimates is the standard error. Nonsampling errors are data errors that stem from any source other than sampling error, such as data collection errors and data-processing errors.
Standard errors can be used to measure the precision with which an estimate from a particular sample approximates the expected result of all possible samples. The chances are about 68 out of 100 that an estimate from the survey differs from a complete population figure by less than the standard error. The chances are about 90 out of 100 that this difference is less than 1.6 times the standard error. Statements of comparison appearing in NCS publications are significant at a level of 1.6 standard errors or better. This means that, for differences cited, the estimated difference is less than 1.6 times the standard error of the difference. To assist users in ascertaining the reliability of NCS series, standard errors or relative standard errors for NCS estimates are available online.
The ECI, ECEC, and benefits publications all use some variation of balanced repeated replication (BRR), a methodology employed to estimate the standard error. The procedure for BRR entails first partitioning the sample into 120 variance strata composed of a single sampling stratum or clusters of sampling strata, and then splitting the sample units in each variance stratum evenly into two variance primary sampling units (PSUs). Next, half-samples are chosen, so that each contains exactly one variance PSU from each variance stratum. Choices are not random, but are designed to yield a “balanced” collection of half-samples. For each half-sample, a “replicate” estimate is computed with the same formula for the regular, or “full-sample,” estimate, except that the final weights are adjusted. A total of 120 replicates are used in this process. If a unit is in the half-sample, its weight is multiplied by (2 – k); if not, its weight is multiplied by k. For all NCS publications, k = 0.5, so the multipliers are 1.5 and 0.5.
The BRR estimate of standard error with R half-sample replicates is
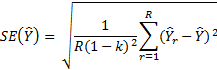
where
the summation is over all half-sample replicates r = 1,...,R,
![]() is the rth half-sample replicate estimate, and
is the rth half-sample replicate estimate, and
![]() is the full-sample estimate.
is the full-sample estimate.
Percent relative standard error data are provided alongside estimates in NCS ECEC publications, which display the standard error as a percentage of the full-sample estimate.
The percent relative standard error is given by
%RSE ![]() )/
)/![]() .
.
Data collection and processing errors are mitigated primarily through quality assurance programs that include the use of data collection reinterviews, observed interviews, computer edits of the data, and a systematic professional review of the data. The programs also serve as a training device to provide feedback to field economists, or data collectors, on errors and the sources of errors that can be remedied by improved collection instructions or computer-processing edits. Extensive training of field economists is conducted to maintain high standards in data collection.
Once estimates of compensation cost changes, of wage and compensation cost levels, or of benefit provisions are produced, the estimates are verified, or validated. The focus of the verification at this stage is a comparison of the estimates with their expected values, which are based on economic conditions; recent trends in similar data; and values prevalent in the recent past as broken out by industry, occupation, bargaining status, region of the country, type of compensation, and other characteristics. Anomalies, such as wage changes outside the historical range, are identified, reviewed, and explained. Estimates are reviewed to ensure respondent confidentiality and specified statistical reliability. Estimates that meet this criteria are designated as “fit for use” and published.
Reliability of the ECI estimates
To assist users in evaluating the reliability of indexes, standard errors for ECI estimates, excluding seasonally adjusted series, are available.
Reliability of the ECEC estimates
To assist users in evaluating the reliability of ECEC estimates, relative standard errors are available for News Release (TXT) (PDF) and Supplemental Private Industry (TXT) (PDF) tables.
Reliability of the benefits estimates
To assist users in evaluating the reliability of benefit estimates, standard errors are available for incidence estimates.