The Linked Employment Cost Index: a first look and estimation methodology

Currently, BLS calculates ECI estimates by using a modified Laspeyres methodology, which is a cell-link update procedure.² In this article, we compare current modified Laspeyres (cell-link) estimates with Linked ECI estimates (based on an index-linking approach), showcasing that these estimates are numerically equivalent. We begin by describing the ECI conceptual framework and the methodologies used for calculating the modified Laspeyres ECI and the Linked ECI. Then, we present our analysis and results for the comparison of index estimates.³ Finally, because indexes that are well suited for one purpose may be ill suited for another, we identify limitations of the Linked ECI, allowing data users to assess whether the new index suits their needs.

Conceptual framework

The ECI is a quarterly measure of changes in compensation costs, which include employer costs for wages, salaries, and employee benefits.⁴ Because the calculation of the ECI assumes that the industrial and occupational composition of employment has not changed since the prior reference period, the index is a fixed-weight, or modified Laspeyres, index. The employment weights for an industry–occupation cell are held constant from the base period in order to reflect changes in compensation costs that are free from changes in employment. BLS estimates the ECI for nonfarm private industry workers and for state and local government workers, excluding federal government workers, private household workers, the self-employed, and workers in the agricultural sector. The National Compensation Survey (NCS) draws a sample of establishments from which it collects data on employer-provided wages, salaries, and employee benefits.⁵ These data, which reflect information for the pay period that includes the 12th day of the reference month, are used to produce the quarterly ECI estimates.

The ECI is also one of the Principal Federal Economic Indicators designated by the Office of Management and Budget. As an important compensation indicator used as an inflationary measure for the U.S. economy, it is closely watched by employers, policymakers, the Federal Reserve, and many other stakeholders. The Federal Reserve uses ECI data to evaluate the labor market effects of its monetary policy, while employers use these data to adjust employee pay and benefits in order to stay competitive. In addition, the ECI includes locality data on compensation costs for 15 major metropolitan areas, providing information that could help businesses and employees decide whether to move to a certain location.

The ECI has undergone several improvements since its inception.⁶ When the index was first introduced in June 1976, it provided data only on private industry wages and salaries. Five years later, in June 1981, BLS started to publish estimates for total compensation (which includes employee benefits besides wages) and for civilian workers and state and local government workers. More recently, in 2008, locality ECI estimates for 15 major metropolitan areas were first published, providing data users with a more geographically detailed picture of compensation costs.

These and other improvements have been focused on publication coverage and sampling rather than estimation methodology. By contrast, the introduction of the Linked ECI would mark a methodological change. Currently, under the modified Laspeyres methodology, BLS publishes separate estimates for different categories of workers (all civilian workers, private industry workers, and state and local government workers) and for different compensation data elements (total compensation, wages and salaries, and employee benefits).

Current estimation methodology for the modified Laspeyres ECI

Currently, BLS calculates the modified Laspeyres ECI with a standard formula for calculating a wage index, which is similar to a total compensation index:

where i denotes the 765 ECI basic cells, CW_i,t is the cost weight of cell i at time (quarter) t, and CW_i,0 is the cost weight of cell i at time (quarter) 0.

The cost weights for the modified Laspeyres ECI for quarter t and time 0 can be calculated, respectively, as follows:

In equations (2) and (3), E_i,b is the fixed employment of cell i for the base quarter b, W_i,t is the compensation of cell i for quarter t (estimated by using the quarterly rates of change since time 0), and  is the compensation of cell i at time 0 (estimated from the sample observations at time 0).

The average compensation of cell i for quarter t is estimated with the following formula:

where r_i,t is the rate of change in compensation between quarter t − 1 and quarter t for cell i and is based on the matched quotes (jobs) between quarter t − 1 and quarter t. This rate can be calculated as

where is the average compensation of cell i for quarter t (estimated from the sample observations in quarter t).

Rearranging equations (1) through (5) by using the average compensation term allows the computation of the modified Laspeyres ECI:

Basic cells for the modified Laspeyres ECI are created for industry and occupational groups sampled from the NCS. There are 765 total cells defined by 9 occupational groups and 85 industry groups, which consist of 59 private industry groups, 13 industry groups from state government, and 13 industry groups from local government. The current modified Laspeyres ECI also has a chaining component (at the cell level), which is reflected in the following formula:

The cost weight for total compensation equals the sum of the cost weights for wages and salaries and the cost weights for total benefits.⁷

Estimation methodology for the Linked ECI

This section provides an overview of the estimation methodology used to calculate the Linked ECI. This is accomplished through an illustrative example that uses a dataset containing information on wages from sampled establishments and quotes. The data from this dataset represent the most recent collection quarter.

The initial variables used in the example are shown in table 1 and are defined as follows:⁸

• Establishment—the business from which data were collected (e.g., a textile mill)
• Quote (job)—the job at the establishment from which data were collected (e.g., a sewing machine operator)
• Industry—the type of industry associated with the establishment
• Average hourly rate—the basic hourly wage paid to the employee
• Weight—the weight associated with the quote, determining how much of the estimate the quote will represent (calculated through sampling and nonresponse adjustment factors like benchmarking)

In this example, we calculate a Linked ECI estimate for wages within the textiles industry. For this reason, all jobs outside the textiles industry (those for establishment 113 in table 1) are excluded from the estimation (see table 2). After identifying the jobs relevant to the estimation of interest, we produce a new variable called “cost weight.” In this example, the cost weight, shown in the last column of table 2, equals the average hourly rate multiplied by the weight.⁹

In the next step of the estimation, we sum the cost weights across the relevant industry. Calculated with this method, the total cost weight for the current collection period, CQCW, is 164,820.5. The total cost weight for the prior period, PQCW, is assumed to be 163,331.0. (In principle, the calculation of PQCW is similar to the calculation of CQCW and involves the summation of cost weights across the relevant industry for the prior quarter.)

To calculate the current-period Linked ECI, we divide the current-period cost weight by the prior-period cost weight and multiply the quotient by the prior-period index:

where CQI is the current-period index and PQI is the prior-period index.

For this example, we assume that the value of the prior-period index is 100.82. Substituting this value and the values of the total cost weights (presented earlier) in equation (8), we arrive at the following calculation: 

This example may not make obvious the “linking” aspect of the estimation. However, this aspect becomes clear when one considers the following statement:

where P₂QI is the index from two quarters ago and P₂QCW is the total cost weight from two quarters ago. Equation (9) can be iterated for all previous quarters, back to the base quarter. Each calculation of an index is based on the previous quarter’s index, giving the estimate its chainlike quality:

Besides calculating Linked ECI estimates, BLS calculates their variances by using Fay’s balanced repeated replication.¹⁰ This method involves producing different half samples from the full sample for the statistic of interest. A half sample selected from the full sample is weighted up, and the other half sample (the one not selected) is weighted down. A new statistic is produced from these reweighted half samples. This process is repeated several times for different orientations of the half samples taken from the full sample. The variance of an index is then calculated by using the statistics created from the replicates, with the following equation:

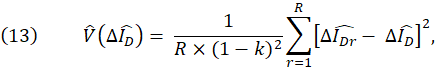
where  is the estimate of the variance of the percent change of index I for domain D; R is the number of replicates; k is a constant, where 0 ≤ k < 1; D is the domain of interest (estimation cell);  is the estimate of the percent change of index I for domain D for replicate r; and  is the estimate of the percent change of index I for domain D based on the full sample.

As in the calculation of the modified Laspeyres ECI, standard errors are calculated only for the 3- and 12-month percent changes in index estimates. The example presented earlier focuses only on producing an estimate for one industry, but for the purposes of estimation, the filtering out of jobs can be expanded to the production of a wide range of estimates by including various industries, occupations, ownerships, and other characteristics. These different combinations of characteristics are called basic cells, which are listings of all estimates that can be produced. In addition, as stated previously, many different forms of compensation can be used to calculate cost weights and generate estimates.

In 2002, BLS introduced the Chained Consumer Price Index for All Urban Consumers (C-CPI-U), which complements two other cost-of-living indexes, the CPI-U and the CPI for Urban Wage Earners and Clerical Workers (CPI-W). Previous research by Michael K. Lettau, Mark A. Loewenstein, and Steven P. Paben has suggested that, unlike the CPI, the ECI is insensitive to the aggregation formula used for its estimation.¹¹ However, the relevancy of the weights used in the estimation decreases as the ECI estimates get far from their base period. The Linked ECI addresses this issue by directly linking the current-period index with the prior-period index.

Analysis and results

This section compares preliminary Linked ECI estimates with those already published for the modified Laspeyres ECI. The comparison covers 8 years of data—from December 2013 to December 2021—because December 2013 was the most recent reweight period for the modified Laspeyres ECI. The calculation of the modified Laspeyres ECI estimates is based on 2012 employment weights and uses employment counts from the BLS Occupational Employment and Wage Statistics program. Linked ECI estimates are computed by using the methodology discussed in the previous section. Here, they are prepared mainly as national estimates for all industries and occupational groups for which modified Laspeyres ECI estimates are already available. Moreover, we compute Linked ECI estimates for both wages and salaries and total benefits, in addition to total compensation costs.

Comparing the modified Laspeyres ECI national estimates with the Linked ECI national estimates shows that, for all industries and all occupational groups in all three ownership groups (civilian workers, private industry workers, and state and local government workers), these estimates do not differ significantly. Tables 3a and 3b present index values and differences between the modified Laspeyres ECI and the Linked ECI for all workers, by ownership type. (The Linked ECI is rebased for comparability with the modified Laspeyres ECI; see appendix.)

As shown in table 3b, for the period from December 2013 to December 2021, the mean absolute percent difference in index estimates for total compensation of civilian workers is 0.03 percent. The largest absolute index difference for total compensation of civilian workers is 0.12 index point, and the smallest is 0.01 index point. For the same reference period, the mean absolute percent difference is 0.03 percent for private industry workers and 0.02 percent for state and local government workers.

Table 4 presents analysis results for the components of total compensation—wages and salaries and total employee benefits—allowing an examination for any differences in index estimates for these components. For wages and salaries, one can observe a relatively larger absolute percent difference for all private industry workers, with a mean value of 0.06 percent. For the same component, the lowest difference, 0.01 percent, is observed for all state and local government workers. For total employee benefits, the difference for state and local government workers is relatively larger, at 0.03 percent.

Further analysis at the industry level reveals relatively large differences between the two indexes for some private sector industries. These industries include leisure and hospitality, wholesale trade, and financial activities. The differences appear in later rather than earlier cycles of the comparison period. (See table 5.) The largest differences are observed between the quarter ending in March 2017 and the quarter ending in December 2021. A test for statistical significance comparing the 3- and 12-month percent changes for the modified Laspeyres ECI with those for the Linked ECI indicates that these changes do not differ significantly.¹²

For the leisure and hospitality industry, table 5 reveals relatively large differences between the two indexes for two quarters in 2020 and for all quarters in 2021. These differences are due mainly to differences in wages. The leisure and hospitality industry was notably affected by the coronavirus disease 2019 (COVID-19) pandemic in both 2020 and 2021, the years for which the differences between the two indexes are observed.¹³ However, statistical tests indicate that, for both 3- and 12-month percent changes, the differences between the two indexes are not statistically significant. Notable differences between index estimates are also observed for the wholesale trade industry, the finance and insurance industry, and the financial activities industry. These industries are characterized by incentive-based pay (a potential contributing factor to index differences), which is pay that includes wages and salaries that are at least partially based on productivity payments, such as production bonuses, commissions, piece rates, or other types of incentives based on production, sales, or output.

Charts 1 and 2 show, respectively, 3- and 12-month percent changes in the modified Laspeyres ECI and Linked ECI estimates. The data are for total compensation of civilian workers and are not seasonally adjusted. As seen in the charts, the 3- and 12-month percent changes for the Linked ECI track closely with the corresponding percent changes for the modified Laspeyres ECI. Of the 32 reference periods for 3-month percent changes, 11 periods exhibit slight differences between the two indexes, but there is no absolute difference larger than 0.1 percentage point. In the case of 12-month percent changes, the incidence of differences between the estimates is lower, with 6 out of 29 reference periods exhibiting absolute differences of 0.1 percentage point. In general, the two indexes tend to move in the same direction for both 3- and 12-month percent changes in total compensation for civilian workers.

But does the story change for different ownerships? Chart 3 shows 3-month percent changes in index estimates for total compensation for all private industry workers. Like the charts for all civilian workers, this chart shows that the Linked ECI estimates track closely with the modified Laspeyres ECI estimates. For the covered period, there are 10 reference periods for which the Linked ECI estimates differ from the modified Laspeyres ECI estimates. In nine of those periods, the absolute difference between the estimates is 0.1 percentage point, and in one period, the difference is 0.2 percentage point. For 22 reference periods, the difference is zero.

In the case of wages and salaries and total benefits, the modified Laspeyres ECI and Linked ECI estimates also track closely with each other for different ownerships. Chart 4 presents 3-month percent changes in index estimates for wages and salaries for all private industry workers. As shown in the chart, the estimates for the two indexes differ in 13 out of 32 reference periods, with the absolute difference in all instances being 0.1 percentage point. In nearly all cases, the direction of growth for the two estimates is the same.

Lastly, chart 5 presents 3-month percent changes in index estimates for total benefits for state and local government workers. Although the absolute differences between the modified Laspeyres ECI and Linked ECI estimates for this series never exceeds 0.1 percentage point, there are 17 instances of such differences. However, statistical tests using standard errors corresponding to those instances indicate that none of the differences are statistically significant, and the same result holds for 12-month percent changes.

Typically, the standard errors for the Linked ECI are slightly larger than the standard errors for the modified Laspeyres ECI. In the case of 3-month percent changes in index estimates for total compensation of civilian workers, there are 24 instances (out of 32 estimates produced since March 2014) in which the standard error for the Linked ECI is larger than the standard error for the modified Laspeyres ECI. In the case of 12-month percent changes in index estimates for total compensation of civilian workers, there are 25 instances (out of 29 estimates produced since December 2014) in which the standard error for the Linked ECI is larger than the standard error for the modified Laspeyres ECI.

Tables 6 and 7 show the standard-error distributions for 3-month percent changed in, respectively, the modified Laspeyres ECI and the Linked ECI. Although the tables present rounded figures, they clearly indicate that the standard errors of the estimates for the two indexes are similar, with those for the Linked ECI being slightly larger than those for the modified Laspeyres ECI. The modified Laspeyres ECI estimates have a higher concentration in the “< 0.2” standard-error category, and the Linked ECI estimates have a higher concentration in the “0.2” standard-error category (in both cases, the difference in percentages is roughly the same). For the other categories, the difference in percentages never exceeds 1 percentage point.

Tables 8 and 9 present the standard-error distributions for 12-month percent changes in, respectively, the modified Laspeyres ECI and the Linked ECI. As shown in the tables, the results in this case are similar to those for 3-month percent changes. For the “0.2” standard-error category, the modified Laspeyres ECI and the Linked ECI are within 0.5 percentage point of each other. The two estimates differ more clearly in the “< 0.2” and “0.3” standard-error categories. The modified Laspeyres ECI estimates have a higher concentration in the “< 0.2” standard-error category, and the Linked ECI estimates have a higher concentration in the “0.3” standard-error category. Again, these results imply that, in general, the Linked ECI has higher standard errors.

Because of its larger standard errors, the Linked ECI might be seen as a diminishment from the modified Laspeyres ECI. However, there are a few factors to consider. First, for both 3- and 12-month percent changes in index estimates, the difference between the modified Laspeyres ECI and the Linked ECI is usually small. In the case of estimates for total compensation of civilian workers, the difference never exceeds 0.1 percentage point. For the same estimates, the difference in standard errors is always less than or equal to 0.1 percentage point. Hence, while the relative difference between standard errors might be large, the absolute difference is not.

Second, a statistical test at the 5-percent significance level across all published estimates and all quarters indicates that the 3- and 12-month percent-change estimates for the modified Laspeyres ECI do not differ significantly from the corresponding percent-change estimates for the Linked ECI. Because the differences between these estimates are often so small (< 0.1 percentage point) and the standard errors of the estimates are often higher than those differences (> 0.1 percentage point), any comparative statements claiming statistically significant differences between the modified Laspeyres ECI and the Linked ECI (within the same quarter) will fail a statistical test with a low significance level.

Conclusion

The analysis results presented in this article suggest that the preliminary estimates for the Linked ECI track closely with the currently published estimates for the modified Laspeyres ECI. In most cases, the absolute percent differences between these estimates are less than 0.1 percent. At the 95-percent confidence level, no 3- or 12-month percent-change estimates differ significantly from each other. This is because, in most cases, the differences between the percent-change estimates are less than 0.1 percentage point, while the differences between the standard errors of those estimates are often slightly greater than 0.1 percentage point. Hence, the Linked ECI and the modified Laspeyres ECI are numerically equivalent.

Producing Linked ECI estimates as a replacement for currently published ECI estimates provides several benefits. First, the Linked ECI offers a straightforward method for index computation. Moreover, because this computation is based on an index-linking approach, it enables a more direct method for implementing potential sample-design changes and offers greater flexibility in calculating standard errors. It also simplifies the process of index reweighting, allowing a change in the compensation definition without the need to wait for a reweight period.

Moving forward, BLS is interested in receiving feedback from data users about the value of calculating Linked ECI estimates and using them as a replacement for the currently published modified Laspeyres ECI estimates. One consideration in assessing this value may involve the frequency of reweighting. Currently, employment weights are updated every 10 years. A possible adoption of the Linked ECI will simplify the reweight process, but reweighting also has the potential to disrupt historical continuity. Given these considerations, BLS will consider any additional feedback from stakeholders to ensure that the frequency of reweighting accurately reflects the slow-moving shifts in the employment mix.¹⁴

Appendix: Rebasing

Rebasing the Linked ECI ensures that the index is comparable to the modified Laspeyres ECI.¹⁵ The procedure involves multiplying Linked ECI and modified Laspeyres ECI estimates and dividing the product by 100. Here, rebasing of the Linked ECI uses the value of the modified Laspeyres ECI for December 2013, the base period, ensuring that the two indexes are equal in that period. The formula for rebasing is given by

where the variable Modified Laspeyres ECI₀ represents the modified Laspeyres ECI at Linked ECI base period 0, and the variable Linked ECI_t represents the Linked ECI at period t.