An official website of the United States government
 United States Department of Labor
United States Department of Labor

Crossref 0
Projections overview and highlights, 2020–30, Monthly Labor Review, 2021.
Labor force and macroeconomic projections overview and highlights, 2022–32, Monthly Labor Review, 2023.
BLS defines full employment as an economy in which the unemployment rate equals the nonaccelerating inflation rate of unemployment (NAIRU), no cyclical unemployment exists, and GDP is at its potential. The full-employment assumption links BLS projections to an economy running at full capacity and utilizing all of its resources.
The Bureau of Labor Statistics (BLS) develops employment projections of the labor market at the national level covering 10 years into the future. These projections are invaluable for career-planning, training-and-education, and policy-planning purposes. Both jobseekers and those transitioning to a new career are provided valuable information that allows them to prepare for jobs that will be the most in demand. National projections also are valuable inputs for states, assisting them in producing projections that will support the allocation of resources for job training.
The projection process starts with an estimation of the labor force at the 10-year horizon.1 Given the supply of labor, macroeconomic aggregates—including total employment, output, productivity, prices, interest rates, and many other variables—are projected.2 This macroeconomic outlook depends on the assumption that the economy will be at full employment in the projection year. At full employment, unemployment will be at the nonaccelerating inflation rate of unemployment (NAIRU) and output will be at potential.3 In its projections, BLS assumes full employment in order to minimize any effect from cyclical fluctuations, focusing instead on structural changes to the economy. In addition to projecting the macroeconomic outlook, BLS projects employment for hundreds of occupations 4 and industries, as well as output for hundreds of industries.5 These employment and industry output projections are estimated separately from the projections for the macro model 6 and are benchmarked to the macro model’s aggregate projections in order to ensure that BLS publishes a consistent view of the economy at full employment.7
A full-employment economy is associated with potential output: the sustainable-trend growth of output (usually expressed as gross domestic product, or GDP; see figure 1) that occurs while the economy is operating at a high rate of resource use. Actual output may be above or below potential output at any single point in time. An economy that is operating above potential output is said to be overheating, which occurs when aggregate demand exceeds productive capacity—a situation that is unsustainable in the long term. By contrast, being below potential output does not necessarily mean that an economy is in recession: the economy could be expanding, but not yet fully recovered from a recession. In general, the economy bounces back and forth between recessionary and overheating periods, correcting itself when it gets too far from its potential output.

| Year | Potential GDP (billions of chained 2009 dollars) | Actual GDP (billions of chained 2009 dollars) |
|---|---|---|
| 1949 | 2051.7 | 2008.9 |
| 1950 | 2161.2 | 2184.0 |
| 1951 | 2276.6 | 2360.0 |
| 1952 | 2405.5 | 2456.1 |
| 1953 | 2521.3 | 2571.4 |
| 1954 | 2598.9 | 2556.9 |
| 1955 | 2663.5 | 2739.0 |
| 1956 | 2732.7 | 2797.4 |
| 1957 | 2815.4 | 2856.3 |
| 1958 | 2915.7 | 2835.3 |
| 1959 | 3023.3 | 3031.0 |
| 1960 | 3141.5 | 3108.7 |
| 1961 | 3266.5 | 3188.1 |
| 1962 | 3399.2 | 3383.1 |
| 1963 | 3550.1 | 3530.4 |
| 1964 | 3708.1 | 3734.0 |
| 1965 | 3871.5 | 3976.7 |
| 1966 | 4045.4 | 4238.9 |
| 1967 | 4236.7 | 4355.2 |
| 1968 | 4435.9 | 4569.0 |
| 1969 | 4627.8 | 4712.5 |
| 1970 | 4789.0 | 4722.0 |
| 1971 | 4932.6 | 4877.6 |
| 1972 | 5081.5 | 5134.3 |
| 1973 | 5240.3 | 5424.1 |
| 1974 | 5426.6 | 5396.0 |
| 1975 | 5621.3 | 5385.4 |
| 1976 | 5802.2 | 5675.4 |
| 1977 | 5988.0 | 5937.0 |
| 1978 | 6193.2 | 6267.2 |
| 1979 | 6402.6 | 6466.2 |
| 1980 | 6568.5 | 6450.4 |
| 1981 | 6718.2 | 6617.7 |
| 1982 | 6922.4 | 6491.3 |
| 1983 | 7149.2 | 6792.0 |
| 1984 | 7397.6 | 7285.0 |
| 1985 | 7676.0 | 7593.8 |
| 1986 | 7962.4 | 7860.5 |
| 1987 | 8244.0 | 8132.6 |
| 1988 | 8516.9 | 8474.5 |
| 1989 | 8784.8 | 8786.4 |
| 1990 | 9042.8 | 8955.0 |
| 1991 | 9276.7 | 8948.4 |
| 1992 | 9503.8 | 9266.6 |
| 1993 | 9750.7 | 9521.0 |
| 1994 | 10023.1 | 9905.4 |
| 1995 | 10319.0 | 10174.8 |
| 1996 | 10633.8 | 10561.0 |
| 1997 | 11010.3 | 11034.9 |
| 1998 | 11457.0 | 11525.9 |
| 1999 | 11933.6 | 12065.9 |
| 2000 | 12409.4 | 12559.7 |
| 2001 | 12860.6 | 12682.2 |
| 2002 | 13244.6 | 12908.8 |
| 2003 | 13584.7 | 13271.1 |
| 2004 | 13926.0 | 13773.5 |
| 2005 | 14272.7 | 14234.2 |
| 2006 | 14578.7 | 14613.8 |
| 2007 | 14843.6 | 14873.7 |
| 2008 | 15098.3 | 14830.4 |
| 2009 | 15310.3 | 14418.7 |
| 2010 | 15457.0 | 14783.8 |
| 2011 | 15615.8 | 15020.6 |
| 2012 | 15815.5 | 15354.6 |
| 2013 | 16049.4 | 15612.2 |
| 2014 | 16305.7 | 16013.3 |
| 2015 | 16573.4 | 16471.5 |
| 2016 | 16832.8 | 16716.2 |
| Sources: Gross domestic product: U.S. Bureau of Economic Analysis; potential gross domestic product: U.S. Congressional Budget Office. | ||
There are two types of factors that cause employment levels to change: cyclical factors and structural factors.8 Cyclical factors are relatively short-term deviations from potential that have their origin in business cycle fluctuations, the normal up-and-down movements of the economy as it cycles through booms and recessions over time.9 Anticipating long-term cyclical changes is not supported by economic theory. Structural factors are changes that have their origin in long-lasting, often permanent, changes in the economy, such as automation or demographic changes. These types of changes can be anticipated and are likely to matter to BLS users, because they have long-term effects on prospective careers and certain types of policy. The full-employment assumption puts the focus on longer term employment changes that are due to structural factors.
The full-employment assumption works by benchmarking BLS projections to the same objective level of output capacity—full, or 100-percent, capacity—each publication round. Without the same objective level of output capacity linked to employment levels, structural effects can be masked or incorrectly perceived, based on changes in the business cycle. Setting the objective level to full capacity is the means by which BLS projections exclude cyclicality; levels are therefore determined entirely by structural factors. Moreover, consistently using the same level of output capacity has the added advantage of allowing changes to be evaluated between different projection release dates. Such evaluation can reveal how the economy has changed structurally between two periods. A consistent objective benchmark for BLS projections is an important tool for identifying structural changes to the economy and is implemented via the full-employment assumption. Being open and explicit about this methodology helps users interpret projections advantageously and use them accordingly.
The assumption of a full-employment economy in the target projection year can have important implications for the BLS projected growth rates and requires one to take into account current cyclical economic conditions when utilizing growth rates. Large cyclical deviations in the base year of projections can influence growth rates: under such conditions, some of the projected growth will be due to cyclical factors.10 In 2010, the year after the most recent recession ended, a year in which the economy was still well below its potential output, BLS published a projected real GDP growth rate of 3.0 percent over the next decade. However, the projected growth rate of potential GDP from 2010 (published by the U.S. Congressional Budget Office) to 2020 was 2.3 percent. This difference implies that 0.7 percent of the published BLS growth rate could be attributed to cyclical factors in the 2010 base value. (See table 1.)
| Type of GDP | 2010 | Projected, 2020 | Growth rate |
|---|---|---|---|
| Actual | 13,088.0 | 17,512.9 | 3.0 |
| Potential | 14,017.1 | 17,512.9 | 2.3 |
| Note: Potential GDP for 2010 is from U.S. Congressional Budget Office, https://www.cbo.gov/sites/default/files/recurringdata/51137-2011-01-potentialgdp.xls. Sources: U.S. Bureau of Labor Statistics (2010), U.S. Congressional Budget Office. | |||
The same dynamic occurs in individual occupation and industry projections. Current full-employment estimates at individual occupation or industry levels do not exist. Therefore, it is impossible to estimate precisely how much of a growth rate is attributable to cyclicality. However, it is often clear that some cyclical effects are present. Figure 2, from BLS 2010 projections, demonstrates their presence. Both construction and extraction occupations and computer and mathematical occupations had projected growth rates of 2.0 percent from 2010 to 2020. Upon examination of how much lower the percentage of employment was for construction and extraction occupations in the 2010 base projection year compared with 2006, it becomes clear that most, or even all, of the growth is attributable to cyclical factors. Conversely, employment growth in computer and mathematical occupations over the same timeframe appears to be mostly structural.
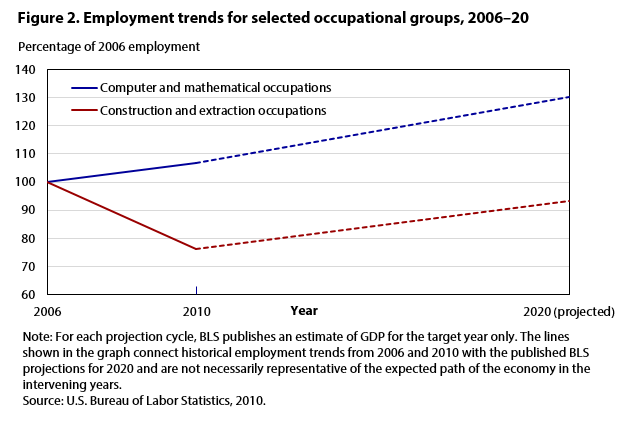
| Occupational group | 2006 | 2010 (percent of 2006 employment) | 2020 (percent of 2006 employment) |
|---|---|---|---|
| Computer and mathematical occupations | 100 | 107 | 130 |
| Construction and extraction occupations | 100 | 76 | 93 |
| Note: For each projection cycle, BLS publishes an estimate of GDP for the target year only. The lines shown in the graph connect historical employment trends from 2006 and 2010 with the published BLS projections for 2020 and are not necessarily representative of the expected path of the economy in the intervening years. Source: U.S. Bureau of Labor Statistics, 2010. | |||
There is no method for directly measuring full employment. Rather, it is a theoretical state within the economy that must be estimated. The idea of full employment has evolved over time. Some unemployment is expected, even desired, in a healthy economy as workers transition to new jobs better matching their skill sets or as they acquire and develop additional skills needed by an employer. These transitions to new jobs and acquisitions of more skills boost the workers’ productivity and the capacity of the economy as a whole. Newer concepts of full employment require some unemployment to temper inflation.
Earlier views, such as those held by Keynes 11 and Beveridge,12 defined full employment as an economy in which the only unemployment was due to workers transitioning from one job to another. This kind of unemployment is known as “frictional unemployment.” Later, “structural unemployment” was added to the definition of full employment. Structural unemployment is the unemployment of workers who currently lack the skills needed by employers. Both frictional unemployment and structural unemployment involve workers who are searching for a job, but, in order to secure a position, workers who are structurally unemployed must do something in addition to just searching. Some must pursue further education or training, while others must relocate to a different geographical area.
Another, more recent view of full employment links employment to inflation. This theory holds that some unemployment is necessary to control inflation. The relationship between the unemployment rate and the rate of inflation is demonstrated in the Phillips curve.13 The level of unemployment required to keep inflation in check is the NAIRU, and most economists today mean the NAIRU when referring to full employment.
Just as an equilibrium exists in the goods-and-services market when supply equals demand, there exists an equilibrium in the labor market when labor supply equals labor demand. At this equilibrium, workers are hired at the prevailing wage and inflation is stable. That is the reason the equilibrium is often referred to as the NAIRU. Shocks to the economy knock the labor market out of equilibrium. If the shock is negative, additional unemployment will emerge as labor demand decreases. Wages generally remain sticky and can’t adjust, amplifying the decrease in labor demand further. If this change in labor demand is temporary, it is considered cyclical unemployment; if it is permanent, the change is known as structural unemployment. Sources of structural changes in labor demand include technology, outsourcing, and demographic changes to the population, among others.
Regardless of whether a shock is temporary or permanent, the U.S. Federal Reserve has some ability to trade higher inflation for lower unemployment in the short run.14 Higher inflation effectively lowers wages so that the demand for labor at the new real wage is higher. This relationship between unemployment and inflation is suggested by the Phillips curve. However, the relationship breaks down over time: individuals begin to expect higher inflation because of increases in prices and wages, and these increases must come at an ever-accelerating rate to support an artificially low unemployment rate. Absent this accelerating inflation, the labor force will return to equilibrium at the NAIRU.
The NAIRU is not a constant for all periods and locations. As discussed earlier, if a shock to labor demand is temporary—a cyclical change—unemployment will return to its preshock level. However, if the shock to labor demand is permanent—a structural change—it will result in a change to the NAIRU and the unemployment level will drift to this new equilibrium. Shocks also affect local economies to varying degrees: some areas have benefited more from recent technological advances and have lower NAIRUs, while others have been hurt more by outsourcing and have higher NAIRUs.15 The degree to which various shocks are structural or cyclical is often a matter of debate, and economists can change their view with time. However, without a doubt, changes to labor demand come from both cyclical and structural factors.
An economy with both the highest level of sustainable employment and stable inflation can still be an economy in which only frictional and structural unemployment exist. Indeed, this is the full-employment assumption that BLS makes: BLS projections assume that the unemployment rate is equal to the NAIRU (implying that inflation is consistent with the Federal Reserve’s target value) and any existing unemployment is frictional or structural.
In addition to including the NAIRU, recent full-employment views link a full-employment economy to potential output. Full employment and a corresponding value for potential output cannot be measured directly. Instead, a number of methods are available for estimating historical values and projecting future values. This section summarizes the most common methods.
Growth accounting methods model output as a production function incorporating the various factors affecting output. Input factors often are adjusted to their potential. One common method for adjusting inputs to their potential uses the relationship between inflation and output (Okun’s law), as well as estimates of the NAIRU. The most popular growth accounting model is the Solow model, which attributes GDP growth to growth in labor, capital, and technological progress. A labor productivity growth model is favored by those who believe that capital is too difficult to measure accurately. This kind of model ignores capital and estimates output as a function of labor and labor productivity alone. Growth accounting methods assume that the magnitude of the contribution that each factor makes to growth remains the same over time.16
Statistical filtering techniques, such as the Hodrick–Prescott filter, extract from the data underlying trends that represent an economy’s full employment or potential output. The drawback to these techniques is that the trend extracted is not benchmarked to any external measure of capacity. Consequently, the estimates obtained are not necessarily consistent with stable inflation. In addition, they are subject to end-of-sample problems, in which the trend becomes more responsive to temporary fluctuations in the data toward the end of a sample.17
Systems of equations can be specified that estimate variables such as output, employment, and inflation together. Although more complex than either growth accounting methods or statistical filtering techniques, these systems allow the contributions of different factors to GDP to vary over time. Systems of equations often include multivariate time series models, such as vector autoregression (VAR).
For its macroeconomic projections, BLS uses a system of equations that constitute a structural econometric model of the U.S. economy. The software involved is licensed from Macroeconomic Advisers (MA), LLC. The system includes a growth model, as well as a VAR that is used for capital projections. BLS uses its own labor force outlook and its own assumptions about total factor productivity (TFP) and the NAIRU as an input into the MA model. Labor interacts with capital and a TFP residual to produce output. Therefore, output is determined not just on the basis of labor but also on that of capital services and potential TFP.18
Because capital is a factor in potential output, including it is pertinent to the model, despite the difficulty that arises in measuring it. Capital stock measures the value of capital in the economy; capital services, which stem from capital stocks, measure the contribution of capital to the production process. Using a capital stock measure weights two pieces of capital the same if they have the same market value, even if their contributions to production are unequal. Because it is the contribution to production that influences both output and the amount of labor necessary to produce that output, a measure of capital services is preferred.
TFP is any growth that is not attributable to capital services or labor. TFP is often taken as a measure of an economy’s technological change,19 although other factors, such as economies of scale and increased education in the labor force, can influence TFP. BLS has projected output on the basis of TFP’s historic trend, assuming that this trend approximates potential TFP. A growing body of research indicates that current TFP, and therefore likely potential TFP, is lower than it was in previous decades.20 As a result, BLS places more weight on recent values.
Deciding what input goes into the MA model is just as important as the model itself, to ensure that the projections obtained are as reasonable as possible. Inputs are adjusted on the basis of (1) current economic research, (2) putting them through alternative BLS models to determine a range of projections, and (3) comparisons with models from other government agencies. Projections that take account of the information gleaned from these studies are then compared against the currently specified MA model. Key exogenous data in the model, including the NAIRU and TFP, are overwritten when BLS deems other values more appropriate.
Although BLS is not limited to any one internal model, the most commonly used model is a
Y = AL0.7K0.3,
where Y = output, A = TFP, L = labor, and K = capital.
The model itself is relatively straightforward. However, identifying what to include for each of the input factors—TFP, labor, and capital—is not. Each input can be estimated any number of ways, all of which likely introduce some degree of measurement error. The labor input has the most objective measurements, but it is still necessary to determine which is most appropriate: employment levels, average hours worked, or some combination of the two. To estimate potential labor input, the NAIRU or other measures of utilization 21 can be used. One BLS approach is
L* = awh* × [(1 – NAIRU) × (lfpr* × cnp)],
where L* = potential labor input, awh* = potential average weekly hours, NAIRU = nonaccelerating inflation rate of unemployment, lfpr* = potential labor force participation rate, and cnp = civilian noninstitutional population.
Capital is more difficult to measure, in part because of the subjectivity of depreciation and, more importantly, the differences in the various types of capital. Capital services indexes offer an easy way of approximating capital, and extending their trends is an appropriate method for projecting capital in the future. Still, it should be noted that these indexes introduce their own set of measurement issues. One is that trends can be estimated in various ways; BLS uses a piecewise linear regression to make its estimations.22
Like capital, TFP indexes exist and provide a good starting point for projecting potential TFP. Unlike capital, however, which is largely a function of investment during previous periods, potential TFP is determined largely by an economy’s technological change and is more subject to sudden shocks as the pace of technological advances increases or decreases. Determining if and how future potential TFP is likely to deviate from its past trend is thus important.
Alternative models, as well as comparisons with other government agencies, are important for a number of reasons. Grounded in economic theory, alternative models allow for a comparative result against which the MA model can be evaluated. Through these models, BLS is able to gain a better understanding of the U.S. economy and the interactions between different variables—an understanding that allows for subsequent sensitivity analysis. Comparisons with outside sources act as a qualitative check confirming that the results are sound.
Like all projections, those produced by BLS are dependent on underlying assumptions about the future. On the basis of how BLS projections are used, such as for career-planning, training-and-education, and policy-planning purposes, structural changes are more important than cyclical changes. Accordingly, BLS projections contain no cyclical deviations; rather, a full-employment assumption is used that projects only structural changes to the economy. Although other definitions of full employment exist, BLS defines full employment as an economy in which the unemployment rate equals the NAIRU, no cyclical unemployment exists, and GDP is at its potential.
The full-employment assumption links BLS projections to an economy running at full capacity and utilizing all of its resources. This—output at 100 percent of capacity—gives users an objective expectation regarding the part of the business cycle on which a particular projected level is based. Because projections are consistently benchmarked to this same objective level, users are provided a means to interpret what is best for them. It is important that interpretations of BLS growth rates be applied in the context of current economic conditions. Although the full-employment assumption ensures that no cyclicality is present in projected levels, some cyclical effects may remain in projected growth rates, depending on the base year of the BLS publication.
In sum, whereas BLS always assumes an economy at full employment, other assumptions affecting projections are subject to change. Factors such as the labor force outlook, the NAIRU, TFP, and capital services are monitored, evaluated, and changed on the basis of current research—research that takes place both within BLS and through comparisons with other agencies. These assumptions ensure that BLS projections both incorporate long-term structural changes to the economy and reflect current conditions and expectations for the future. The assumptions apply to projections of the aggregate economy, as well to projections of the products benchmarked to it: projections of GDP at a detailed level, and industry and occupational employment projections.
Kevin S. Dubina, "Full employment: an assumption within BLS projections," Monthly Labor Review, U.S. Bureau of Labor Statistics, November 2017, https://doi.org/10.21916/mlr.2017.30
1 For data on the labor force, see “Employment projections: labor force projections” (U.S. Bureau of Labor Statistics, October 24, 2017), https://www.bls.gov/emp/data/labor-force.htm.
2 For data on the aggregate economy, see “Employment projections: aggregate economy” (U.S. Bureau of Labor Statistics, October 24, 2017), https://www.bls.gov/emp/data/aggregate-economy.htm.
3 Hereafter, potential output.
4 For data on occupational employment, see “Employment projections: occupational employment projections” (U.S. Bureau of Labor Statistics, October 26, 2017 ), https://www.bls.gov/emp/data/occupational-data.htm.
5 For data on industry output and employment, see “Employment projections: industry output and employment projections” (U.S. Bureau of Labor Statistics, October 24, 2017), https://www.bls.gov/emp/data/industry-out-and-emp.htm ; for data on interindustry relationships, see “Employment projections: inter-industry relationships (Input–Output Final Demand matrix)” (U.S. Bureau of Labor Statistics, October 24, 2017), https://www.bls.gov/emp/data/input-output-matrix.htm.
6 For additional information about employment projection methods, see BLS handbook of methods (U.S. Bureau of Labor Statistics), chapter 13, “Employment projections,” https://www.bls.gov/opub/hom/pdf/homch13.pdf.
7 The most recent BLS projections appear in T. Alan Lacey, Mitra Toossi, Kevin Dubina, and Andrea Gensler, “Projections overview and highlights, 2016–26,” Monthly Labor Review, October 2017, https://doi.org/10.21916/mlr.2017.29.
8 Frictional factors are a third type; however, we can effectively ignore them because changes in frictional factors that affect unemployment levels can be attributed to structural or cyclical factors.
9 See Mark Thoma, “Will there be a ‘new normal’ for unemployment?” MoneyWatch, cbsnews.com, November 11, 2009, http://www.cbsnews.com/news/will-there-be-a-new-normal-for-unemployment/.
10 BLS could estimate current full-employment levels, rather than using actual employment, in determining growth rates. However, this approach would misconstrue the true level of job growth and likely cause confusion among users. In addition, it often takes years to identify structural changes to the economy. Therefore, using an estimate of current full-employment levels would introduce an additional source of error because perceived cyclical changes at the time may later be identified as structural changes.
11 See John Maynard Keynes, The general theory of employment, interest and money (London: Macmillan and Co., Limited, 1936).
12 See Sir William Beveridge, Full employment in a free society (London: The New Statesman and Nation and Reynolds News, 1944), http://lib-161.lse.ac.uk/archives/beveridge/9A_79_Full_employment_in_a_free_society.pdf.
13 See Kevin D. Hoover, “Phillips curve,” The concise encyclopedia of economics, Library of Economics and Liberty, http://www.econlib.org/library/Enc/PhillipsCurve.html.
14 See Jeffrey M. Lacker and John A. Weinberg, Inflation and unemployment: a layperson’s guide to the Phillips curve (Richmond, VA: Federal Reserve Bank of Richmond, 2006), https://www.richmondfed.org/~/media/richmondfedorg/publications/research/annual_report/2006/pdf/article.pdf.
15 See Marilyn Geewax, “Why some still can't find jobs as the economy nears ‘full employment,’” All Things Considered, National Public Radio, January 31, 2016, http://www.npr.org/2016/01/31/464856256/why-some-still-cant-find-jobs-as-the-economy-nears-full-employment.
16 See “CBO’s method for estimating potential output: an update” (Congressional Budget Office, August 2001), https://www.cbo.gov/sites/default/files/107th-congress-2001-2002/reports/potentialoutput.pdf.
17 See “A summary of alternative methods for estimating potential GDP” (Congressional Budget Office, March 2004), https://www.cbo.gov/sites/default/files/108th-congress-2003-2004/reports/03-16-gdp.pdf.
18 See “Revisions to CBO’s projection of potential output since 2007” (Congressional Budget Office, February 2014), https://www.cbo.gov/sites/default/files/113th-congress-2013-2014/reports/45150-PotentialOutput-OneColumn.pdf.
19 See Richard G. Lipsey and Kenneth Carlaw, “What does total factor productivity measure?” (Vancouver: Simon Fraser University, January 18, 2001), http://www.csls.ca/ipm/1/lipsey-e.pdf.
20 See Robert J. Gordon, “Slower U.S. growth in the long- and medium-run,” NBER Reporter 2015 Number 1: Research Summary (Cambridge, MA: National Bureau of Economic Research), http://www.nber.org/reporter/2015number1/gordon.html.
21 For additional information about alternative measures of labor underutilization, see “Alternative measures of labor underutilization for states, third quarter of 2016 through second quarter of 2017 averages,” Local Area Unemployment Statistics (U.S. Bureau of Labor Statistics, July 28, 2017), https://www.bls.gov/lau/stalt.htm.
22 For a comprehensive view of an internal BLS model, including formulas, see Maggie Woodward, “A simple model for estimating potential output,” in Proceedings of the 19th Federal Forecasters Conference: the value of government forecasts (U.S. Bureau of Labor Statistics, September 27, 2012), pp. 25–33, http://www.va.gov/healthpolicyplanning/ffc/pandp/ffc2012.pdf. For further discussion of piecewise linear regressions, see Robert S. Pindyck and Daniel L. Rubinfeld, Econometric models and economic forecasts, 4th ed. (Boston: Irwin/McGraw Hill, 1998).